Electrostatics Formulas

1. Fundamental forces of nature

• Gravitational
• Electromagnetic,
• Nuclear,
• Weak.

Relative strength 1 : 10³⁶ : 10³⁹ : 10¹⁴
Charge is quantised, the quantum of charge is e = 1.6 × 10^-19 C.
Charge is conserved, invariant, additive

2. Coulomb’s law

$\overrightarrow{\mathrm{F}}=\mathrm{K}\frac{{\mathrm{q}}_1{\mathrm{q}}_2}{{\mathrm{r}}^2}\hat{\mathrm{r}}$
K = $\frac{1}{4\pi {\epsilon }_0}$ = 9 × 10⁹$\frac{{\mathrm{Nm}}^2}{{\mathrm{C}}^2}$
ε₀ = 8.854 × 10^-12$\frac{C^2}{N{m}^2}$
= Permittivity of free space
$\frac{\epsilon }{{\epsilon }_0}$ = ε_r = Relative permittivity or dielectric constant of a medium.
$\overrightarrow{\mathrm{E}}=\frac{\mathrm{Kq}}{{\mathrm{r}}^2}\hat{\mathrm{r}}$

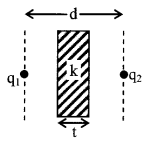
Note: – If a plate of thickness t and dielectric constant k is placed between the j two point charges lie at distance d in air then new force
$\mathrm{F}=\frac{{\mathrm{q}}_1{\mathrm{q}}_2}{4\pi {\epsilon }_0(\mathrm{d}-\mathrm{t}+\mathrm{t}\sqrt{\mathrm{k}}{)}^2}$
effective distance between the charges is
d’ = (d – t + t$\sqrt{\mathrm{k}}$)

3. Intensity of electric field

$\overrightarrow{\mathrm{E}}$ = Force on a unit positive charge = $\frac{\overrightarrow{\mathrm{F}}}{{\mathrm{q}}_0}$ N/C or V/m.
Due to a point charge q intensity at a point of positive vector $\overrightarrow{\mathrm{r}}$
$\overrightarrow{\mathrm{E}}=\frac{\mathrm{Kq}}{{\mathrm{r}}^2}\hat{\mathrm{r}}$

4. Electric potential

Work done against the field to take a unit positive charge from infinity (reference point) to the given point.
V_P = – ${\int }_{\mathrm{\infty }}^P\overrightarrow{E}\cdot \overrightarrow{dr}\text{ volt }$
Due to a point charge q, potential
V =K $\frac{q}{r}$ volt

5. Principle of superposition

Resultant force due to a number of charges
$\overrightarrow{\mathrm{F}}={\overrightarrow{\mathrm{F}}}_1+{\overrightarrow{\mathrm{F}}}_2+\dots ..+{\overrightarrow{\mathrm{F}}}_{\mathrm{n}}$
Resultant intensity of field
$\overrightarrow{\mathrm{E}}={\overrightarrow{\mathrm{E}}}_1+{\overrightarrow{\mathrm{E}}}_2+\dots ..{\overrightarrow{\mathrm{E}}}_{\mathrm{n}}$
Resultant potential V = V₁ + V₂ + … + V_n

6. Relation between $\overrightarrow{\mathrm{E}}$ and V

$\overrightarrow{\mathrm{E}}$ = – grad V = – $\overrightarrow{\mathrm{\nabla }}V=-\frac{\partial V}{\partial r}\hat{r}$
In cartesian coordinates
$\overrightarrow{\mathrm{E}}=-[\hat{\mathrm{i}}\frac{\partial \mathrm{V}}{\mathrm{dx}}+\hat{\mathrm{j}}\frac{\partial \mathrm{V}}{\partial \mathrm{y}}+\hat{\mathrm{k}}\frac{\partial \mathrm{V}}{\partial \mathrm{z}}]$

7. Electric flux

Treating area element as a vector
dΦ = $\overrightarrow{\mathrm{E}}\cdot \overrightarrow{\mathrm{ds}}$, Φ = ${\int }_s\overrightarrow{\mathrm{E}}\cdot \overrightarrow{\mathrm{ds}}$ volt – metre

8. Gauss’s theorem

Total outward flux through a closed surface = (4πK) times of charge enclosed
or Φ = $\oint \overrightarrow{\mathrm{E}}\cdot \overrightarrow{\mathrm{ds}}=4\pi \mathrm{K}\sum \mathrm{q}=\frac{1}{{\epsilon }_0}\mathrm{\Sigma }\mathrm{q}$

9. Intensity and potential due to a non-conducting charged sphere

${\overrightarrow{\mathrm{E}}}_{\text{out }}=\frac{1}{4\pi {\epsilon }_0}\frac{\mathrm{Q}}{{\mathrm{r}}^2}\hat{\mathrm{r}},{\mathrm{E}}_{\text{out }}\propto \frac{1}{{\mathrm{r}}^2}$
${\overrightarrow{\mathrm{E}}}_{\text{surface }}=\frac{1}{4\pi {\epsilon }_0}\frac{\mathrm{Q}}{{\mathrm{R}}^2}\hat{\mathrm{r}}$
${\overrightarrow{\mathrm{E}}}_{\text{inside }}=\frac{1}{4\pi {\epsilon }_0}\frac{\mathrm{Q}}{{\mathrm{R}}^3}\overrightarrow{\mathrm{r}},{\mathrm{E}}_{\text{inside }}\propto \mathrm{r}$

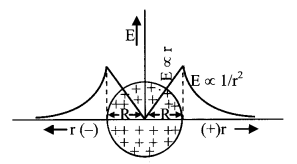
V_out = K $\frac{Q}{r}$, V_surface = K $\frac{Q}{r}$
and V_inside = $\frac{\mathrm{KQ}(3{\mathrm{R}}^2-{\mathrm{r}}^2)}{2{\mathrm{R}}^3}$
V_centre = $\frac{3}{2}\frac{\mathrm{KQ}}{\mathrm{R}}$ = 1.5 V_surface

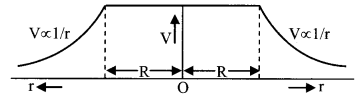
10. Intensity and potential due to a conducting charged sphere

Whole charge comes out on the surface of the conductor.
${\overrightarrow{\mathrm{E}}}_{\text{out }}=\frac{1}{4\pi {\pi }_0}\frac{\mathrm{Q}}{{\mathrm{r}}^2}\hat{\mathrm{r}}$
${\overrightarrow{\mathrm{E}}}_{\text{surface }}=\frac{1}{4\pi {\epsilon }_0}\frac{\mathrm{Q}}{{\mathrm{R}}^2}\hat{\mathrm{r}}$
${\overrightarrow{\mathrm{E}}}_{\text{inside }}=0$

V_out = K$\frac{Q}{r}$
V_surface = K$\frac{Q}{R}$
V_inside = K$\frac{Q}{R}$ (Constant)

11. Electric potential and field intensity due to a charged ring

On axis
V = $\frac{KQ}{{(R^2+x^2)}^{1/2}}$
$\overrightarrow{\mathrm{E}}=\frac{\mathrm{KQx}}{{({\mathrm{R}}^2+{\mathrm{x}}^2)}^{3/2}}\hat{\mathrm{x}}$

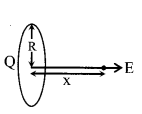
(x is the distance of the point on the axis from the centre)
At centre E = 0, V = $\frac{\mathrm{KQ}}{\mathrm{R}}$
Note: – If charged ring is semicircular then E.F. at the centre is
$\frac{2\mathrm{K}\lambda }{\mathrm{R}}=\frac{\mathrm{Q}}{2{\pi }^2{\mathrm{R}}^2{\epsilon }_0}$
and potential V = $\frac{\mathrm{KQ}}{\mathrm{R}}$

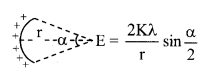
12. Electric field intensity due to very long (∞) line charge

$\overrightarrow{\mathrm{E}}=\frac{2\mathrm{K}\lambda }{\mathrm{r}}\hat{\mathrm{n}}=\frac{1}{2\pi {\epsilon }_0}\frac{\lambda }{\mathrm{r}}\hat{\mathrm{n}}$
$\hat{\mathrm{n}}$ is a unit vector iionpjd to line charge.

13. Electric field intensity due to a charged sheet having very large (∞) surface area

$\overrightarrow{\mathrm{E}}$ = 2πK σ $\hat{\mathrm{n}}$ (constant)
σ → charge of unit cross section

14. Electric field intensity due to an infinite charged conducting plate

$\overrightarrow{\mathrm{E}}$ =4πKσ $\hat{\mathrm{n}}=\frac{\sigma }{{\epsilon }_0}\hat{\mathrm{n}}$(constant)
σ → charge of unit surface area

15. Electric dipole

Two equal and opposite point charges separated by a small distance. Dipole moment $\overrightarrow{\mathrm{p}}=\mathrm{q}\overrightarrow{\mathrm{d}}$. Direction of $\overrightarrow{\mathrm{p}}$ is from -q to + q.

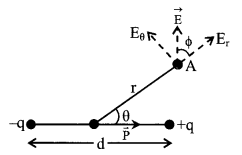
Potential at a point A (r, θ)
V = $\frac{\mathrm{Kqd}\cos \theta }{{\mathrm{r}}^2}$
V = $\frac{\mathrm{Kp}\cos \theta }{{\mathrm{r}}^2}=\mathrm{K}\frac{\overrightarrow{\mathrm{p}}\cdot \overrightarrow{\mathrm{r}}}{{\mathrm{r}}^3}$

Electric field intensity

E = $\frac{\mathrm{p}}{4\pi {\epsilon }_0{\mathrm{r}}^3}\sqrt{1+3{\cos }^2\theta }$
E_r = 2K$\left(\frac{\mathrm{p}\cos \theta }{{\mathrm{r}}^3}\right)$
E_θ = K $\left(\frac{p\sin \theta }{r^3}\right)$
E = $\sqrt{{\mathrm{E}}_{\mathrm{r}}^2+{\mathrm{E}}_{\theta }^2}$
On axis θ = 0, E_r = E = $\frac{2\mathrm{kp}}{{\mathrm{r}}^3}$

On equatorial θ = $\frac{\pi }{2}$, E_θ = E = $\frac{\mathrm{Kp}}{{\mathrm{r}}^3}$
Angle between E.F. at point A and x – axis is (θ + Φ)
where tan Φ = $\frac{1}{2}$ tan θ

16. Dipole in an electric field

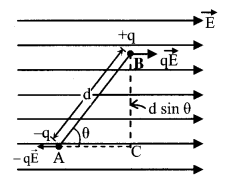
In a uniform field F_net = 0, (No translatory motion)
Torque $\overrightarrow{\tau }=\overrightarrow{\mathrm{p}}\times \overrightarrow{\mathrm{E}}$ or τ = pE sin θ
Potential energy of dipole
U = – $\overrightarrow{\mathrm{p}}\cdot \overrightarrow{\mathrm{E}}$
(dipole perpendicular to field is taken as reference state). Work done in rotating the dipole from θ₁ to θ₂.
W = U₂ – U₁ = pE (cos θ₁ – cos θ₂)
Time period of oscillation of electric dipole in uniform E.F.
T = 2π$\sqrt{\frac{I}{P.E}}$;
I = moment of inertia

17. Equilibrium of charged soap bubble

For a charged bubble
P_ext + P_elct. = $\frac{4\mathrm{T}}{\mathrm{r}}$
For P_ext = 0, P_elct. = $\frac{4\mathrm{T}}{\mathrm{r}}$
or $\frac{{\sigma }^2}{2{\epsilon }_0}=\frac{4T}{r}$

Electric field on surface
E_surface = ${\left(\frac{8\mathrm{T}}{{\epsilon }_0\mathrm{r}}\right)}^{1/2}$
Potential on surface
V_surface = ${\left(\frac{8\mathrm{Tr}}{{\epsilon }_0}\right)}^{1/2}$

18. Energy density in electric field

U = $\frac{1}{2}$ ε₀E² (J/m³)

19. When small drops of charge q forms a big drops of charge Q

Q = nq, R = r n^1/3
V_big =n^2/3 V_small

20. Electric break-down or electric strength

Max. electric field can be created in the given medium.
For air E_max = 3 × 10⁶ V/m

Seek help on various concepts taking the help of Formulas provided on the trusted portal Onlinecalculator.guru and clear all your ambiguities.